Science news last week included a finding that the answer is no. Why should I care if the universe is symmetric? And why should I read about the evidence? Since the beginning of scientific cosmology (it began with Einstein, of course, in a footnote to his exposition of General Relativity) — since the beginning of cosmology, everyone in this field has assumed that the universe is extraordinarily symmetric. Perfectly symmetric, in a sense I’ll explain. Why have they all bet the farm on such perfection? And how much of the story of the Big Bang and 13.7 billion years and dark energy and accelerating expansion — how much of this is cast in doubt if it turns out the universe is not symmetric?
Einstein’s gravitational field equations, or General Relativity, were a triumph for science. It was the first time that really complex, edgy mathematics found application to the real world, and what was explained was the nature of space and time themselves. General Relativity changed the culture of science, with the idea that some very abstract, impossible-to-visualize concepts could actually be the best description for our very world, the one we live in.
Newton’s gravity (1665) was elegant and quite simple. 1/r^2 is a computation that most high school algebra students can understand. The electromagnetic equations of Maxwell (1865) were elegant and less simple, The magnetic field comes from changes in the electric field in a perpendicular direction. Remarkably, the electric field can be generated by changes in the magnetic field in the same way. It’s accessible to second year calculus students.
Einstein’s field equations (1915) were a quantum leap in complexity beyond Maxwell. (Not a pun — there is no quantum mechanics in these equations.) Before you can even begin to understand them, you have to swallow the hard (and hard-to-believe) fact that our idea about rectilinear coordinates is quite arbitrary; North-South & East-West & Up-Down could be replaced by any arbitrary assignment of numbers to each point, so long as it is continuous. I don’t mean just rotating the coordinates to Northeast-Southwest, for example. I mean drawing wiggly (but smooth) lines every which way and using that for your coordinate system. Crazy as it seems, Einstein tells us it doesn’t matter — the calculation of gravity and motion is just the same, no matter how you assign numbers to the points in space. Time, too, could be assigned numbers that change very rapidly, and then much slower a second later. If that’s how you want to measure time, it’s OK with Albert.
Then you have to imagine how to translate your mathematical description of space and time into the one you’ll use a second later when you’re at a different point in your travels. You have to define the most natural and continuous connection between the coordinates you used one second ago and the coordinates you’re using now. This turns out to involve tensors. The terms in Einstein’s equations are 4-dimensional blocks of numbers, like a matrix squared. 4 by 4 by 4 by 4 numbers, 64 numbers all told arranged in a hypercube. This is R, a condensed form of the Riemann-Christoffel tensor, which is the thing that mass-energy bends out of shape, and which is also the guide which every planet follows as it finds its way.
The equation doesn’t look so complicated, but that’s because of the tremendous complexity subsumed in the symbol R. For the more mathematically inclined: The Einstein Field equations represent 64 nonlinear partial differential equations in 64 unknowns.
Here’s the punchline: The Einstein equations are way too complicated for anyone to solve. I don’t just mean you can’t write down a function that is a solution. I mean you can’t even solve it numerically using a computer. Not with a fancier computer that you imagine, not with a computer the size of the universe. These equations give you no idea what the solutions look like. 107 years after Einstein wrote down the equations, we can’t say what they predict.
Einstein’s Field Equations are a proof that God has a sense of humor. “You want to know what makes the clock tick? You want to be able to predict the future? OK — here it is. This is my rule book...Go at it!”
The equations of ordinary, non-relativistic quantum mechanics are even more impossible to solve (if you’ll forgive my use of a comparative with an absolute adjective), but that’s a story for another day.
There are exceptions, of course. When gravitational fields are weak, the equations of General Relativity are indistinguishable from Newton’s equations for gravity. So that’s what astrophysicists use when they make numerical simulations of the formation and clustering of galaxies.
The above simulation was made with Newton’s equations, not Einstein’s. So it is good science so long as gravity is weak in the part of the universe that is simulated.
To model the universe as a whole, we cannot assume that the gravitational field is weak. But there is one other circumstance under which we can solve Einstein’s equations, and that is spherical symmetry. The collapse of a spherical ball of gas into a black hole can be computed exactly. A spinning sphere or black hole can be handled adequately. The 64 differential equations reduce to one.
For the universe as a whole, if it is limitless, then the idea of a sphere doesn’t apply directly. The equivalent conditions you need in order to be able to solve the equations are called “homogeneity and isotropy”. Homogeneity means that the universe is the same everywhere. Isotropy applies the further stipulation that the uniform stuff of the universe is not rotating.
So here’s the dirty little secret of cosmology: Ever since Einstein, the equations for the expansion and evolution of the universe have been solved under the assumption that the universe is homogeneous and isotropic. We theoretical physicists make that assumption, not because we look at the sky and find that it is homogeneous and isotropic, but because without that assumption we don’t know how to solve the equations.
In fact, when I was in high school, cosmology was considered a speculative science, widely disparaged by physicists. The reason was that the subject was top-heavy with fancy theory, without a basis in observation. The only relevant observation was Hubble’s Law, which says that the further out in the sky we look for galaxies, the more they are redshifted, indicating that galaxies are receding from us and the speed of recession is proportional to the distance from us.
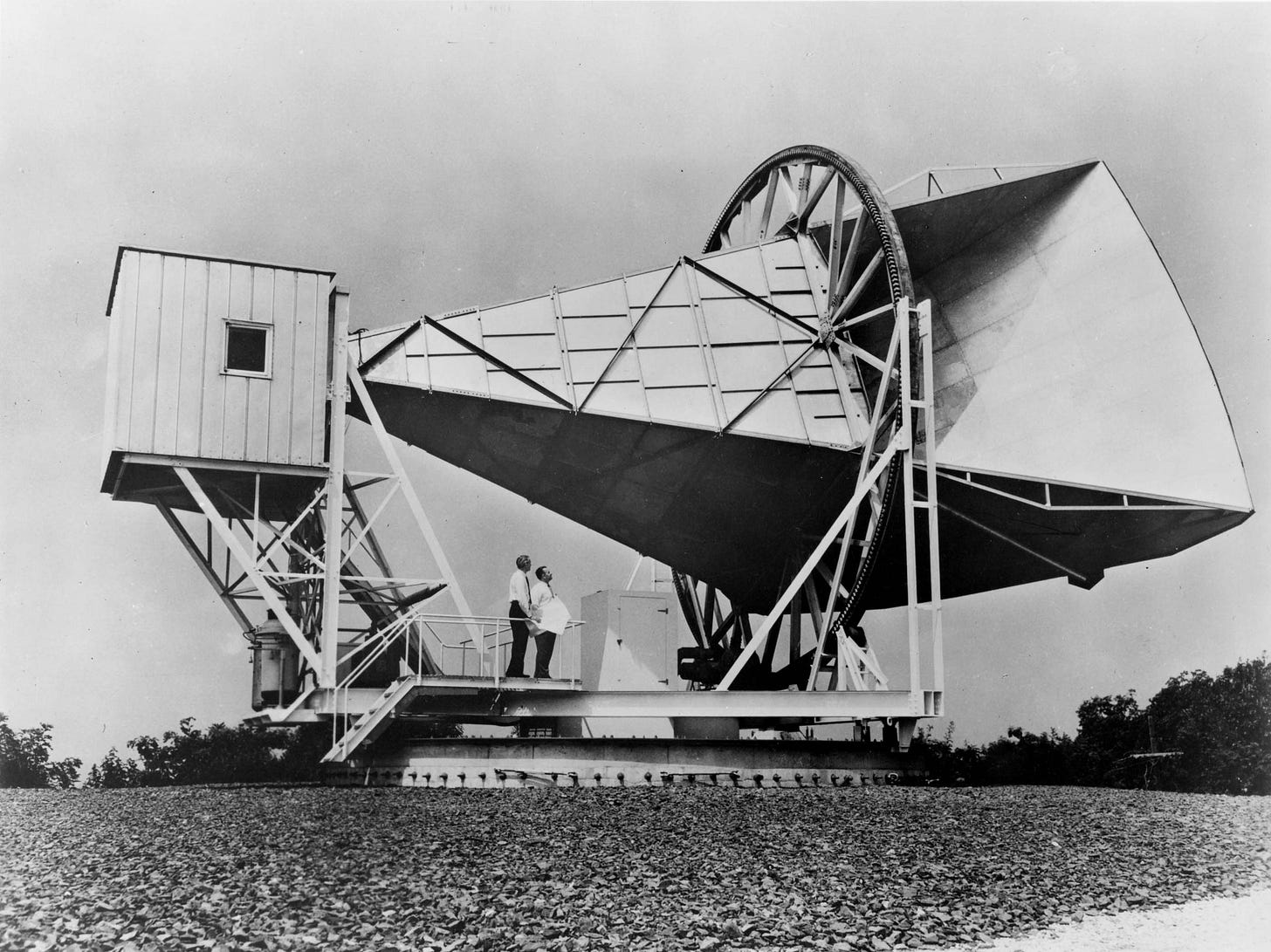
The observation that turned cosmology into a respectable science was the 3 degree microwave background, discovered serendipitously by Penzias and Wilson in 1965, as they were trying to get rid of the residual noise they found in their radio antenna.
This one observation was enough to enable physicists to extrapolate back in time, how hot the universe was during the first 3 minutes of the Big Bang, and that temperature was enough to be able to ask what kinds of nuclear chemistry should have been taking place among the electrons, neutrons, and protons flying around during those first few minutes. The prediction was that the universe should be about ¾ hydrogen and ¼ helium. When we point spectrographic telescopes at some of the oldest stars, this prediction is confirmed — ¾ hydrogen and ¼ helium. This was the triumph that made cosmology a respectable science.
The thirty-five years following 1965 saw a flowering of cosmology, based on advances in computer science. Computer-controlled telescopes could take pictures and spectra of millions of galaxies, and so maps could be made of the distribution of galaxies. At the same time, computers became powerful enough to predict the motions of gas and dust, the formation of gas clouds that turned into galaxies and stars and black holes. Matching up the theoretical computations with the observations was a big challenge — as big as problems in physics can get. It was an exciting time to be an astrophysicist. We were making 3D maps of the universe and trying to understand them.
Then the simple story of a Big Bang yielding the right mix of H and He and then expanding uniformly developed wrinkles. The big picture seemed to work, but not the details. The universe was clumpier than we can account for. There were clusters of galaxies with vast holes in between, and then clusters of clusters (“superclusters”) and it still isn’t clear if superclusters are clustered on a larger scale yet. There wasn’t enough mass to explain the formation of galaxies or the speed that they’re rotating, so the models needed a lot of invisible mass. Invisible isn’t so bad, but the matter couldn’t be normal electrons, neutrons, and protons because that would mess up our understanding of nuclear chemistry in the first three minutes and the 3:1 ratio of hydrogen to helium. So a new name was invented for some mysterious stuff; we may not know what “dark matter” is, but we know that it is nothing we have seen on earth, including the largest particle accelerators. In 1997, two groups of astronomers using very different methods found evidence that the expansion of the universe is accelerating — the opposite of what you’d expect if the matter in all these stars and galaxies was pulling each other back. So a form of negative gravity needed to be added to the equations, and this is was named “dark energy”, again an empty label for something hypothesized but completely unknown.
The universe is rotating
The hero of this story is Michael Longo, a physicist from University of Michigan, who reported a simple observation in 2007 that could have been made decades earlier, if anyone would have thought to do it.
For a pinwheel galaxy, you can tell its direction of rotation just by looking at it. Longo collected telescope images of the northern sky and asked his students to record the rotational directions of 15,000 galaxies. There were more left-handed galaxies in the northern sky, and correspondingly more right-handed galaxies in the southern sky. There is a net angular momentum that is the same in both directions.
Our part of the universe is rotating.
“Homogeneous and isotropic” — a hundred-year-old assumption of cosmology, has been shown to be a fiction.
(Longo’s work was peer-reviewed and published in 2011 in Physics Letters. Lior Shamir automated the analysis with computers that could look at more galaxies, and he confirmed Longo’s observation in 2012.)
This discovery has received so little attention in the intervening years precisely because it is so revolutionary, so to speak. Everything we have theorized about the structure of our universe should be re-thought from the ground up. It’s too much to absorb, so we just go on as if nothing had happened.
By the by, I’m reminded of the front page headlines in the New York Times in December, 2017. On December 16, the Times announced that UFOs are real after all, and that the US Navy has visual and radar evidence of flying saucers doing things that our 21st century physics says should be impossible. On December 17, the same NYTimes went on to report that “Homeowners have it too good, says the tax bill.” When everything is changed, life goes on as if nothing is different. We are only human, and we can’t pivot on a dime when what we always assumed turns out to be wrong.
This summer, a much fancier computer algorithm was used by Oliver Philcox (Columbia University) to analyze positions of galaxies rather than their visual orientation. He confirmed that the universe has a preferred direction, but curiously did not reference Michael Longo. Katie McCormick wrote up the finding for Quanta Magazine, but also seems unaware of Longo. But now the subject has some respectability, and perhaps some astrophysicists will acknowledge that they have been solving the wrong equation.
Since 2015, astrophysicists have been looking for their keys under the streetlight, by solving the only version of Einstein’s Field Equations that they know how to solve. Now we know that the universe they have been modeling is not our universe.
For me, the moral of this story is that we must look for what is right under our noses, invisible to us because it doesn’t jive with our deeply-held premise about reality.